This tutorial is designed to give users a better understanding of logic gates and how to set up your own logic circuits for purposes such as converting binary into a language readable by a 7-segment display. I will begin by giving a brief overview on the basic skills required such as a simple understanding of boolean algebra, the types of logic gates, truth tables and karnaugh maps.

Boolean Algebra

Boolean Algebra is a form of algebra consisting of only two possible answers. True or false. In boolean algebra there is no such thing as 2, 3, 4, or -3, -2, -1. Only True and false, or in the case of binary, 1 and 0. In boolean algebra there is no such thing as division or subtraction. The set of rules governing boolean algebra are different to that of normal algebra. Here are some of those rules:

1 + 0 = 1

1 + 1 = 1

1 * 0 = 0

1 * 1 = 1

As you can see from these rules, it is evident that multiplication has the same effect that an AND gate would have (only outputs TRUE if all inputs are TRUE). Secondly, we can see that addition has the same function as an OR gate (outputs TRUE as long as at least one of the inputs is TRUE).

Lastly it is important to note that in boolean algebra, when a variable has an apostrophe to the right of it, it is called the complement of that variable. For example, take A. If we change A to A', it is now the complement of A. If:

A = 1, then A' = 0

if

A = 0, then A' = 1

There are other ways of displaying a complement. The most common way is to place a horizontal line over the complemented variable. Another less common way is to place a tilde to the left of the variable. Like so ~A.

Logic Gates

(It is important to note that these logic gates assume that the inputs are being given a constant flow of electricity. So in most cases you should not be sending single sparks into the inputs, rather you should be using batteries to power a constant flow of electricity to the inputs. Also, be aware that the presence of electricity is represented by 1, and the absence of electricity is represented by 0.)

Logic gates are essentially the manifestation of boolean expressions in the form of circuitry. Logic gate can be represented in Powder Toy, and they are often used to create logic circuits. There are several types of logic gates. These include:

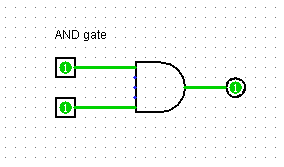
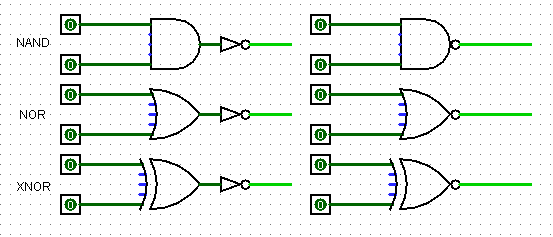
AND gate

An AND gate is a logic gate that will only output TRUE if all inputs are TRUE. If this condition is not met, the output will be FALSE. In this image you can see an AND gate in its typical representation. It has two inputs (on the left) and one output (on the right). Please note that AND gates can have any number of inputs. It is not uncommon to see a 3-input AND gate.

The following save shows a functional AND gate (along with other gates) made in Powder Toy.

https://powdertoy.co.uk/Browse/View.html?ID=96171 (alexthesax)

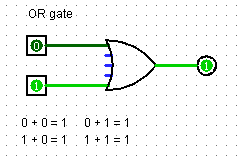
OR gate

An OR gate is a logic gate that will output TRUE as long as at least one of the inputs are TRUE. An OR gate will also output TRUE if both inputs are TRUE. This is the typical representation of an OR gate.

The following save shows a functional OR gate (along with other gates) made in Powder Toy.

https://powdertoy.co.uk/Browse/View.html?ID=96171 (alexthesax)

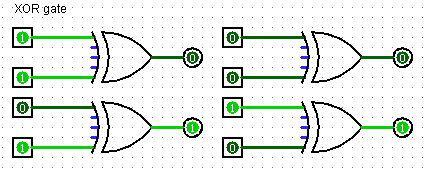
XOR gate

And XOR gate is slightly different to an OR gate. The X stands for exclusive. This means that the XOR gate will only output TRUE if one but not both inputs are TRUE. This is an example of an XOR gate in all of its states.

The following save shows a functional XOR gate (along with other gates) made in Powder Toy.

https://powdertoy.co.uk/Browse/View.html?ID=96171 (alexthesax)

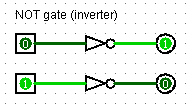
NOT gate (or inverter)

A NOT gate outputs the opposite of the input. For example, if the input was 1, the output would be 0. And vise versa. NOT gates are usually used in conjunction with AND/OR/XOR gates to reverse their functions. NOT gates are also used to represent the complement of a variable. For example if A = 1, then A' = 0. Placing a NOT gate after an AND gate (NAND gate) will represent this, and therefore, the output will always be the complement of A. This is the typical representation of the two possible states for a NOT gate.

The following save shows a functional NOT gate (along with other gates) made in Powder Toy.

https://powdertoy.co.uk/Browse/View.html?ID=96171 (alexthesax)

NAND/NOR/XNOR gates

These gates are simply AND/OR/XOR gates with a NOT gate placed on their outputs. The resulting effect is that if the input was A + B =1(OR gate), it would change to A' + B' =1(NOR). If it was AB = 1 (AND gate) then it would change to A'B' = 1. If it was an XOR gate it would also invert. Note that XOR gates aren't represented in boolean algebra by the common addition or multiplication signs, however XOR is equal to AB' + A'B. This shows the three gates. I will show two ways to represent them (with a bubble on the outputs, and with the full NOT gate. Both mean the same thing).

Truth tables

Now that you have an understanding of the basic boolean algebra concepts as well as their logic gate equivalents, it is time to learn about truth tables. A truth table is a table used to show all of the possible outputs for a given set of inputs. It is also used to dictate the outputs a person would want from every possible combination of inputs. It is possibly the most powerful tool in creating a logic circuit that will do what you want it to do.

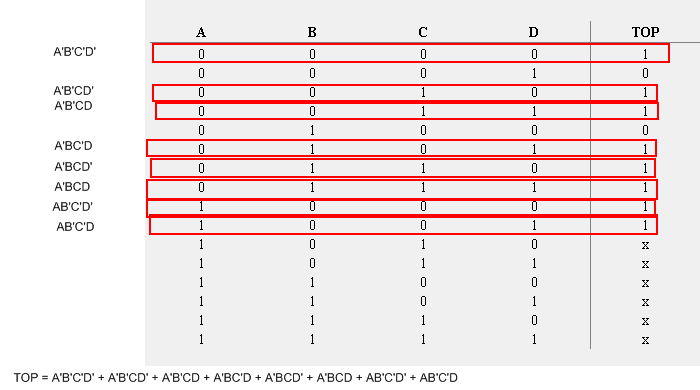
For example, what if we wanted to convert a 4-bit binary number into language readable by a 7-segment display. This means that we need 4 inputs for the binary number that we are attempting to convert. We will name these inputs A,B,C and D. Notice that the inputs were place on the left side of the table in columns. The (none) represents where we will place the outputs that have not yet been defined. As you can see the 4 inputs take up 16 rows in order to show every possible combination of inputs.

Next we will add the outputs. For a binary to 7-segment decoder we would need 7 outputs. 1 output for each segment of the display. Later we will link these outputs to 1 segment each. I labeled the outputs according to the segment they will be attached to (on the 7 segment display)

This shows the segments each output corresponds to.

Next we are going to assign values to each of the outputs. The way we do this is as follows.

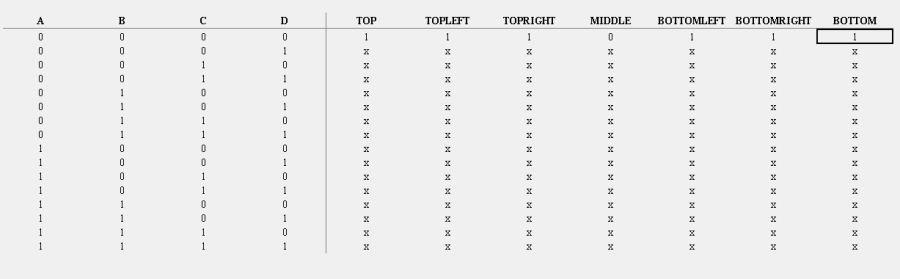
1. We look at row one. The value in the inputs is 0 (A=0,B=0,C=0,D=0). 2. If the inputs were equal to zero, that would mean we would want to display zero on the seven segment display. So we change the value in each output column to 1, except for the value in the column labeled middle, because in representing a zero in a seven segment display, we would need to turn on every segment except for the middle one. If you correspond the values in this image to the image of the 7 segment digit, you will see that we have represented a zero. Assuming that the segments are lit up when a value of 1 is present and turned off when a value of 0 is present.

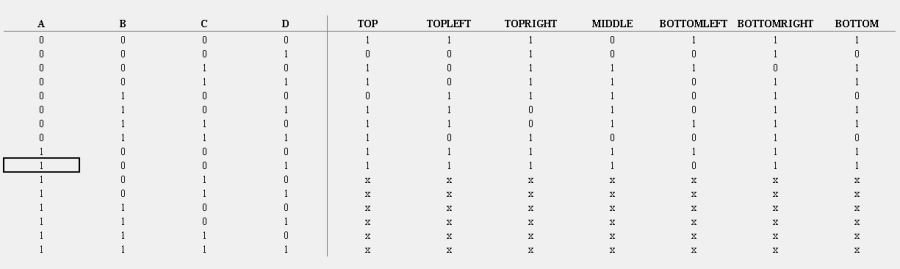
3. We will go down every row, one row at a time and do the exact same thing. Note that we will not need to continue after the tenth row, as for a single digit the highest value displayable is 9. (the values in the rows after A=1, B=0, C=0, D=1 (9) can be left as x, which means "don't care". By doing this we define that the outputs for the inputs 1001 to 1111 don't matter, and they can be either 1 or 0.) However if you were doing a hexadecimal display, you may want to use all of the rows. This is what your table should now look like (for 0-9).

Now we have finished our truth table. We have defined our inputs as A,B,C,D for our 4-bit binary input, and we have defined our outputs which each correspond to a segment on a seven segment display digit. The next step is to convert this table into a logic circuit.

Building the circuit

The next step is to extract the information from the truth table. To do this we will start by looking at each output. For the first output, TOP, if you look down its column you will notice that it is equal to 1 in 8 of the rows. Theses are the rows we are going to focus on.

Deriving the equations

We will focus on the TOP output for now. We are going to generate an equation from TOP. The method is as follows.

1. Look down the TOP column. As you can see, the first 1 is in row one. Take note of the value of the inputs in this row.

2. The inputs for this row are A=0,B=0,C=0 and D=0. Write on a piece of paper, TOP = A'B'C'D'

3. Once again look down the TOP column until you find the next 1. The next 1 is located on row three which has the values A=0,B=0,C=1 and D=0.

4. Add this to your equation like so. TOP = A'B'C'D' + A'B'CD (please note that a value of 0 is defined with the complementary sign, and a value of 1 is not. Also note that A'B'C'D is the same as A' * B' * C' * D'. * is the sign for multiplication.)

5. Now we are going to do the same thing for every other 1 found in the column and add them to the equation. It should look like so. With the finished equation for TOP.

6. The final step, is indeed going to be tedious, but you are going to have to do the same thing for every other output. Don't worry, I have to do it too, so it isn't that hard. Also note that usually it would be far quicker than this, but 7 outputs is a lot of outputs. After about 15 minutes this is the finished group of equations I came up with.

TOP = A'B'C'D' + A'B'CD' + A'B'CD + A'BC'D + A'BCD' + A'BCD + AB'C'D' + AB'C'D

TOPLEFT = A'B'C'D' + A'BC'D'+ A'BC'D + A'BCD' + AB'C'D' + AB'C'D

TOPRIGHT = A'B'C'D' + A'B'C'D + A'B'CD' + A'B'CD + A'BC'D' + A'BCD + AB'C'D' + AB'C'D

MIDDLE = A'B'CD' + A'B'CD + A'BC'D' + A'BC'D + A'BCD' + AB'C'D' + AB'C'D

BOTTOMLEFT = A'B'C'D' + A'B'CD' + A'BCD' + AB'C'D'

BOTTOMRIGHT = A'B'C'D' + A'B'C'D + A'B'CD + A'BC'D' + A'BC'D + A'BCD' + A'BCD + AB'C'D' + AB'C'D

BOTTOM = A'B'C'D' + A'B'CD' + A'B'CD + A'BC'D + A'BCD' + AB'C'D'+ AB'C'D

Building the circuit

Now we have the equations for each output. From this we can build a circuit. Please note that in the future I will be adding a section for simplifying these equations. As this is possibly the most raw unsimplified version possible, and will result in an extremely large and over-complicated logic circuit. But for now, I will just show you how this circuit is made. The method is as follows.

You can do this on paper, in Powder toy or in a program such as Logisim. I am going to be using Logisim, as it is the easiest to immediately layout and convey the method. Logisim is a free program and has an amazing feature which allows you to write a truth table, and it will automatically generate a logic ciruit for you, along with the equations.

1. Layout your 4 inputs and 7 outputs in a similar way to this. Note that I am using Logisim for the sake of clarity.

2. Lets look at the equations. Notice how they are all groups of inputs + groups of inputs. Remember that addition is the same function as an OR gate. We will look at the equation for TOP first. There are 8 seperate groups of 4 inputs.

The equation says TOP = A'B'C'D' + A'B'CD' + A'B'CD + A'BC'D + A'BCD' + A'BCD + AB'C'D' + AB'C'D. This means we need to use an OR gate with 8 inputs and 1 output. If you look at the equation you will notice that TOP does not equal A'B'C'D' * A'B'CD' * A'B'CD * A'BC'D * A'BCD' * A'BCD * AB'C'D' * AB'C'D. If this was TOP's equation, it would mean that TOP would only output TRUE if all 8 of those groups of conditions were met. However luckily the equation states differently. The addition signs in the equation means that TOP = A'B'C'D, TOP also = A'B'CD', TOP also = A'B'CD ect. So we can use an 8 input OR gate to show that all of these conditions don't need to be TRUE for the output to return TRUE, only one of them. So go ahead and place an 8 input OR gate where I have.

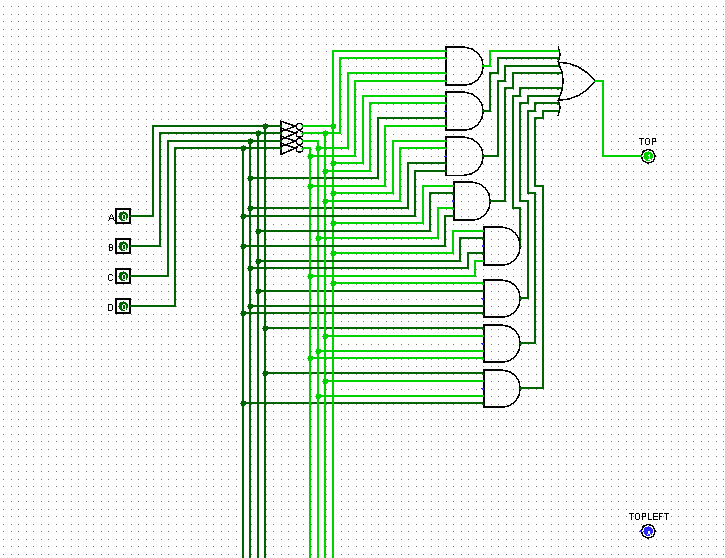
3. Continuing with the TOP output, the next thing we need to adress is the 8 groups of 4 variables. If you look at the diagram, you will see that every input to the OR gate actually corresponds to one of the 4 variable groups. For example, the up most input is supposed to be equal to A'B'C'D'. This group of 4 variables, along with all of the other variable groups, can also be represented as A' * B' * C' * D'. Remember that multiplication is the same as the function of an AND gate. So in order to make the uppermost input to the OR gate equal A'B'C'D', we are going to need to use a 4 input AND gate. The 4 inputs to this AND gate are must consist of the complement of A, the complement of B, the complement of C and the complement of D. How do we achieve this? With a NOT gate.

Let me explain what I have done. I first created a row of wires that travels vertically downwards. These rows represent the inputs A, B, C and D. I then branched each of these inputs off and used NOT gates to change their values to A', B', C' and D'. Notice how the second set of vertical wires is lit up. That is because they are the complement of whatever the input is, in this case the inputs are turned off, and therefore the inverted wires are equal to 1. I created an AND gate and I linked A',B',C' and D' to its inputs. I then linked the output from that AND gate to the top most input for the OR gate.

4. The next step is to do the same thing for the other 7 groups. This is what it should look like after you have finished. (Remember that is an extremely unsimplified version, and later on I will a section on karnaugh maps and equation simplification, which will give you a far smaller circuit.

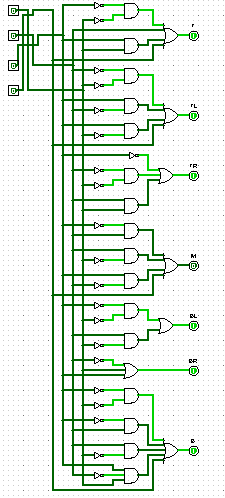
5. Now you need to do the exact same thing you did in steps 2,3 and 4 for every other output. This is what it should look like (this has been completely re-arranged in order to create more space. It will look different, but it won't matter because creating the circuitry for the other 6 outputs is exactly the same method as used for the first output.

This is the finished result after re-arranging. I got rid of the row of complemented inputs and just placed NOT gates where needed to conserve space. The image is so damn long that I had to use another method to save it, which doesn't have colour. I will make a new one soon. Please note that on this image the "1" on the outputs doesn't mean that the output is TRUE. It just seems to be there regardless. In actuality every output is TRUE except for the middle output which is FALSE. This represents zero on a seven segment digit. Although it doesn't show this on the image, where it says every output is 1/TRUE.

Simplification with karnaugh maps (coming soon)

In this section I will show users how to simplify their circuits with algebraic simplification and karnaugh maps. This is an example of how far the binary to 7 segment decoder can be simplified.

(FINISHED SECTION COMING SOON)

--Synergy 12:23, 5 October 2011 (BST)